Mgt 509 Home     Fall 2006     Email to Dr. Lyons       PatLyons Home  

[ Calendar | Photo | Class Participation AI | Application of Ops Mgt | Table of Contents | Search ] 

[ Chapter 1 | 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | 12 | 13 | 14 | 15 | 16 | 17 |  | HW1 | 2 ] 
 [ SJU | TCB | CareerCenter | QueensEvents | COACH | CareerLinks | MBAServices | Internships ] [ ProjectLiberty ]

Ch 9 - Project Management

  1. Introduction
    1. Project (p361)
      1. Def - one-of-a-kind production of a customized product that requires a long completion time (from Ch6, p223).
      2. Examples - constructing a building, developing a new product.
  2. Elements of Project Management (p363)
    1. Project team - organization can be:
      1. Temporary - individuals assigned from different departments within company and then disbanded.
      2. *Permanent - Matrix Organization - two-dimensional structure with members from different functional areas (dimension 1) assigned to various projects (dimension 2).
    2. Project Scheduling - determining (p367):
      1. Activities - tasks which make up the project
      2. Precedent relationships - activities which must be completed before another activity can start
      3. Activity time - time it takes to perform an activity
  3. Gantt Chart
    1. Popular tool for scheduling small projects
    2. Example - Fig 9.4, p368
  4. *Critical Path Method (p371)
    1. Def - Basic Critical Path Method (CPM) - a method to determine the activities which are critical to completing a project on time.
    2. Example Fig 9.8 - building a house, p373 - designate activities with letters not numbers (reduces confusion).

             Activity

        Precedent
        Relationship

        Activity 
        Time (months)
        A - Design house
        B - Lay foundation
        C - Order materials 
        D - Build house
        E - Select paint
        F - Select carpet
        G - Finish work      		    -
         A
         A
         B, C
         B, C
         E
         D, F      		    3
         2
         1
         3
         1
         1
         1
    3. Construct Project Network

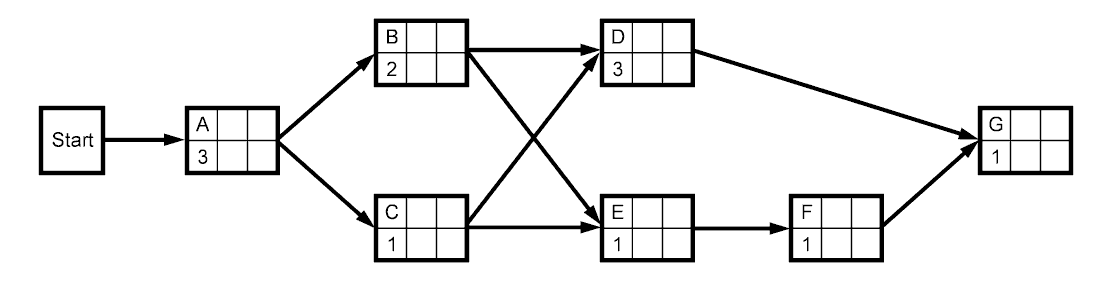
  1. Basic CPM Procedure (p375)
    1. Compute earliest start (ES) time and earliest finish (EF) time for each activity (forward pass)
      1. ES of activities with no precedent relationships = 0
      2. EF for a given activity = its ES + its activity time
      3. ES for a given activity = largest EF of the immediately preceding activities.
    2. Compute latest start (LS) time and latest finish (LF) time for each activity (backward pass)
      1. LF of any final activity = the largest EF
      2. LS for a given activity = its LF - its activity time
      3. LF for a given activity = smallest LS of the immediately succeeding activities.
    3. Compute slack time for each activity: Slack = LS – ES
    4. *Identify the critical path. An activity is on the critical path if its slack time = 0.
          Note: If an activity on the critical path takes longer than estimated, then final date will be affected.
    5. Example - Fig 9.12 (continued)

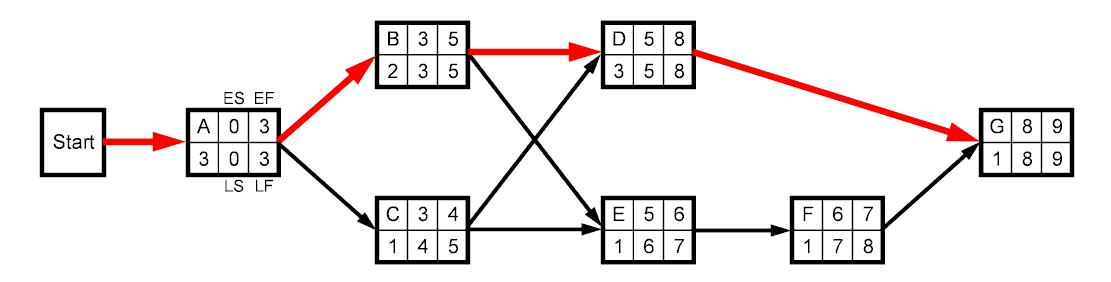
      Activity 

      Slack = LS - ES 

        A
        B
        C
        D
        E
        F
        G    		     0 - 0 = 0
        3 - 3 = 0
        4 - 3 = 1
        5 - 5 = 0
        6 - 5 = 1
        7 - 6 = 1
        8 - 8 = 0

    Critical path: A, B, D, G
    Do assigned HW - Problem 9-8.
    (This page was last edited on August 16, 2006 .)